Toán 10 [SGK Mới] Bài 11: Tích vô hướng của hai vector

[Timeless time]

[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
Chắc suất Đại học top - Giữ chỗ ngay!!

ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.

TÍCH VÔ HƯỚNG CỦA HAI VECTOR​

1. Góc giữa hai vector
Cho hai vector [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{v}[/imath] khác [imath]\overrightarrow{0}[/imath].
Từ một điểm [imath]A[/imath] tuỳ ý, vẽ các vector [imath]\overrightarrow{u} = \overrightarrow{AB}, \overrightarrow{v} = \overrightarrow{AC}[/imath].
Khi đó, số đo góc [imath]BAC[/imath] được gọi là số đo góc giữa hai vector [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{v}[/imath] hay đơn giản là góc giữa vector [imath]\overrightarrow{u}, \overrightarrow{v}[/imath].
Kí hiệu là: [imath](\overrightarrow{u}, \overrightarrow{v})[/imath]
Chú ý:
- Quy ước giữa hai vector [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{0}[/imath] có thể nhận một giá trị tuỳ ý từ [imath]0^\circ[/imath] đến [imath]180^\circ[/imath].
- Nếu [imath](\overrightarrow{u}, \overrightarrow{v}) = 90^\circ[/imath] thì ta nói rằng [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{v}[/imath] vuông góc với nhau, kí hiệu là [imath]\overrightarrow{u} \perp \overrightarrow{v}[/imath] hoặc [imath]\overrightarrow{v} \perp \overrightarrow{u}[/imath]
- Đặc biệt [imath]\overrightarrow{0}[/imath] được coi là vuông góc với mọi vector

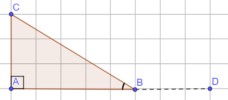
Ví dụ: Cho tam giác $ABC$ vuông tại $A$ và $\hat B = 30^\circ$. Tính $(\overrightarrow{AB}, \overrightarrow{AC}), (\overrightarrow{CA}, \overrightarrow{CB}), (\overrightarrow{AB}, \overrightarrow{BC})$

Giải:

Ta có: - [imath](\overrightarrow{AB}, \overrightarrow{AC}) = \widehat{BAC} = 90^\circ[/imath] - [imath](\overrightarrow{CA}, \overrightarrow{CB}) = \widehat{ACB} =60^\circ[/imath] - [imath](\overrightarrow{AB}, \overrightarrow{BC}) = (\overrightarrow{DB}, \overrightarrow{BC}) = \widehat{DBC} = 150^\circ[/imath]

2. Tích vô hướng của 2 vector
Tích vô hướng của hai vector [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{v}[/imath] là một số, kí hiệu là [imath]\overrightarrow{u}\cdot \overrightarrow{v}[/imath], được xác định bởi công thức sau: [imath]\overrightarrow{u}\cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{u}| \cdot \cos (\overrightarrow{u}, \overrightarrow{v})[/imath]
Chú ý:
- [imath]\overrightarrow{u} \perp \overrightarrow{v} \iff \overrightarrow{u}\cdot \overrightarrow{v} = 0[/imath]
- [imath]\overrightarrow{u}\cdot \overrightarrow{u}[/imath] còn được viết là [imath]\overrightarrow{u}^2[/imath] và được gọi là bình phương vô hướng của vector [imath]\overrightarrow u[/imath]. Ta có: [imath]\overrightarrow{u}^2 = |\overrightarrow{u}| \cdot |\overrightarrow{u}| \cdot \cos 0^\circ = |\overrightarrow{u}| ^2[/imath]

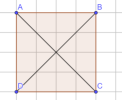
Ví dụ: Cho hình vuông $ABCD$ có cạnh bằng $a$. Tính các tích vô hướng sau: $\overrightarrow{AB} \cdot \overrightarrow{AD}, \overrightarrow{AB} \cdot \overrightarrow{AC}, \overrightarrow{AB} \cdot \overrightarrow{BD}$.

Giải:

- Vì [imath](\overrightarrow{AB}, \overrightarrow{AD}) = 90^\circ[/imath] nên [imath]\overrightarrow{AB} \cdot \overrightarrow{AD} = 0[/imath] Hình vuông có cạnh bằng [imath]a[/imath] nên đường chéo bằng [imath]a\sqrt 2[/imath]. Mặt khác [imath](\overrightarrow{AB}, \overrightarrow{AC}) = 45^\circ, (\overrightarrow{AB} ,\overrightarrow{BD} = 35^\circ[/imath], do đó: - [imath]( \overrightarrow{AB} \cdot \overrightarrow{AC} )= AB \cdot AC \cdot \cos 45^\circ = a \cdot a\sqrt 2 \cdot \dfrac{\sqrt 2}2 = a^2[/imath] - [imath](\overrightarrow{AB} \cdot \overrightarrow{BD}) = AB \cdot BD \cdot \cos 135^\circ = a \cdot a\sqrt 2 \cdot \left(-\dfrac{\sqrt 2}2 \right) = -a^2[/imath]

3. Biểu thức toạ độ và tính chất của tích vô hướng

3.1 Định nghĩa
Tích vô hướng của hai vector [imath]\overrightarrow{u} = (x;y), \overrightarrow{v} = (x',y')[/imath] được tính theo công thức: [imath]\overrightarrow{u}\cdot \overrightarrow{v} = xx'+yy'[/imath]
Nhận xét:
- Hai vector [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{v}[/imath] vuông góc với nhau khi và chỉ khi [imath]xx' + yy' = 0[/imath]
- Bình phương vô hướng của [imath]\overrightarrow{u} (x;y)[/imath] là: [imath]\overrightarrow{u}^2 = x^2 + y^2[/imath]
- Nếu [imath]\overrightarrow{u} \ne 0[/imath] và [imath]\overrightarrow{v} \ne 0[/imath] thì [imath]\cos (\overrightarrow{u}, \overrightarrow{u}) = \dfrac{\overrightarrow{u}\cdot \overrightarrow{v}}{|\overrightarrow{u}| \cdot |\overrightarrow{v}|} = \dfrac{xx' + yy'}{\sqrt{x^2 + y^2} \cdot \sqrt{x'^2 + y'^2}}[/imath]

Ví dụ: Trong mặt phẳng toạ độ $Oxy$, tính tích vô hướng của các cặp vector sau:
a. $\overrightarrow{u} = (2;-3), \overrightarrow{v} = (5;3)$.
b. Hai vector $\overrightarrow{i}, \overrightarrow{j}$ tương ứng với trục $Ox, Oy$.

Giải:
a. Ta có: [imath]\overrightarrow{u}\cdot \overrightarrow{v} = 2 \cdot 5 + (-3) \cdot 3 = 1[/imath]
b. Vì [imath]\overrightarrow{i} = (1;0)[/imath] và [imath]\overrightarrow{j} = (0;1)[/imath] nên [imath]\overrightarrow{i}\cdot \overrightarrow{j} = 1 \cdot 0 + 0 \cdot 1 = 0[/imath]

3.2 Tích chất của tích vô hướng
Với ba vector [imath]\overrightarrow{u}, \overrightarrow{v}, \overrightarrow{w}[/imath] bất kì và mọi số thực [imath]k[/imath] ta có :
- [imath]\overrightarrow{u} \cdot \overrightarrow{v} = \overrightarrow{v} \cdot \overrightarrow{u}[/imath] (tính chất giao hoán)
- [imath]\overrightarrow{u}( \overrightarrow{v} + \overrightarrow{w}) = \overrightarrow{u} \cdot \overrightarrow{v} +\overrightarrow{u} \cdot \overrightarrow{w}[/imath] ( tính chất phân phối)
- [imath]( k \cdot \overrightarrow{ u} ) \cdot \overrightarrow{v} = ( k \cdot \overrightarrow{ u} \cdot \overrightarrow{v} ) = ( k \cdot \overrightarrow{ v} ) \cdot \overrightarrow{u}[/imath]

 

[Timeless time]

GIẢI BÀI TẬP SGK​

4.21. Trong mặt phẳng tọa độ Oxy, hãy tính góc giữa hai vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] trong mỗi trường hợp sau:
a. [imath]\overrightarrow{a} (-3;1), \overrightarrow{b} (2;6)[/imath]
b. [imath]\overrightarrow{a} (3;1), \overrightarrow{b} (2;4)[/imath]
c. [imath]\overrightarrow{b} (-\sqrt 2; 1), \overrightarrow{b} (2;- \sqrt 2)[/imath]

Lời giải:
a.
Ta có: [imath]\overrightarrow{a} \cdot \overrightarrow{b} = (-3) \cdot 2 + 1 \cdot 6 = 0 \implies (\overrightarrow{a}, \overrightarrow{b}) = 90^\circ[/imath]
b.
Ta có: [imath]\overrightarrow{a} \cdot \overrightarrow{b} = 3 \cdot 2 + 1 \cdot 4 = 10[/imath]
[imath]|\overrightarrow{a}| = \sqrt{3^2 + 1^2} = \sqrt{10}, |\overrightarrow{b}| = \sqrt{2^2 + 4^2} = 2\sqrt{5}[/imath]
[imath]\cos (\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|}= \dfrac{10}{\sqrt{10} \cdot 2 \sqrt 5} = \dfrac{1}{\sqrt 2}[/imath]
[imath]\implies (\overrightarrow{a} , \overrightarrow{b}) = 45^\circ[/imath]
c.
Ta có: [imath]\overrightarrow{a} \cdot \overrightarrow{b} = (-\sqrt 2)\cdot 2 + 1 \cdot (-\sqrt 2) = -3\sqrt 2[/imath]
[imath]|\overrightarrow{a}| = \sqrt{( -\sqrt 2)^2 +1^2} = \sqrt 3 , |\overrightarrow{b}| = \sqrt{2^2 + (-\sqrt 2)^2} = \sqrt 6[/imath]
[imath]\cos (\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|}= \dfrac{-3\sqrt 2}{\sqrt 3 \cdot \sqrt 6} = - 1[/imath]
[imath]\implies (\overrightarrow{a} , \overrightarrow{b}) = 180^\circ[/imath]

4.22. Tìm điều kiện của [imath]\overrightarrow{u}, \overrightarrow{v}[/imath] để:
a. [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath]
b. [imath]\overrightarrow{u} \cdot \overrightarrow{v} = - |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath]

Lời giải:
a.
Ta có: [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v} \cdot \cos(\overrightarrow{u}, \overrightarrow{u})[/imath]
Để [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath] thì [imath]\cos(\overrightarrow{u}, \overrightarrow{u}) = 1 \iff (\overrightarrow{u}, \overrightarrow{u}) = 0^\circ[/imath]
[imath]\implies \overrightarrow{u}, \overrightarrow{v}[/imath] cùng hướng
b.
Ta có: [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v} \cdot \cos(\overrightarrow{u}, \overrightarrow{u})[/imath]
Để [imath]\overrightarrow{u} \cdot \overrightarrow{v} = - |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath] thì [imath]\cos(\overrightarrow{u}, \overrightarrow{u}) =- 1 \iff (\overrightarrow{u}, \overrightarrow{u}) = 180^\circ[/imath]
[imath]\implies \overrightarrow{u}, \overrightarrow{v}[/imath] ngược hướng

4.23. Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho hai điểm [imath]A(1;2), B(-4;3)[/imath]. Gọi [imath]M(t;0)[/imath] là một điểm thuộc trục hoành.
a. Tính [imath]\overrightarrow{AM} \cdot \overrightarrow{BM}[/imath] theo [imath]t[/imath]
b. Tính [imath]t[/imath] để [imath]\widehat{AMB} = 90^\circ[/imath]

Lời giải:
a. Ta có [imath]\overrightarrow{AM} = (t-1; -2), \overrightarrow{BM} = (t + 4; -3)[/imath]
[imath]\implies \overrightarrow{AM} \cdot \overrightarrow{BM} = (t - 1)(t+4) + (-2) (-3) = t^2 + 3t + 2[/imath]
b. Để [imath]\widehat{AMB} = 90^\circ[/imath] thì [imath]\overrightarrow{AM} \cdot \overrightarrow{AM} = 0[/imath]
[imath]\iff t^2 + 3t + 2 = 0 \iff \left[\begin{array}{l} t = -1 \\ t = -2 \end{array} \right.[/imath]

 

[Timeless time]

GIẢI BÀI TẬP SGK​

4.21. Trong mặt phẳng tọa độ Oxy, hãy tính góc giữa hai vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] trong mỗi trường hợp sau:
a. [imath]\overrightarrow{a} (-3;1), \overrightarrow{b} (2;6)[/imath]
b. [imath]\overrightarrow{a} (3;1), \overrightarrow{b} (2;4)[/imath]
c. [imath]\overrightarrow{b} (-\sqrt 2; 1), \overrightarrow{b} (2;- \sqrt 2)[/imath]

Lời giải:
a.
Ta có: [imath]\overrightarrow{a} \cdot \overrightarrow{b} = (-3) \cdot 2 + 1 \cdot 6 = 0 \implies (\overrightarrow{a}, \overrightarrow{b}) = 90^\circ[/imath]
b.
Ta có: [imath]\overrightarrow{a} \cdot \overrightarrow{b} = 3 \cdot 2 + 1 \cdot 4 = 10[/imath]
[imath]|\overrightarrow{a}| = \sqrt{3^2 + 1^2} = \sqrt{10}, |\overrightarrow{b}| = \sqrt{2^2 + 4^2} = 2\sqrt{5}[/imath]
[imath]\cos (\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|}= \dfrac{10}{\sqrt{10} \cdot 2 \sqrt 5} = \dfrac{1}{\sqrt 2}[/imath]
[imath]\implies (\overrightarrow{a} , \overrightarrow{b}) = 45^\circ[/imath]
c.
Ta có: [imath]\overrightarrow{a} \cdot \overrightarrow{b} = (-\sqrt 2)\cdot 2 + 1 \cdot (-\sqrt 2) = -3\sqrt 2[/imath]
[imath]|\overrightarrow{a}| = \sqrt{( -\sqrt 2)^2 +1^2} = \sqrt 3 , |\overrightarrow{b}| = \sqrt{2^2 + (-\sqrt 2)^2} = \sqrt 6[/imath]
[imath]\cos (\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|}= \dfrac{-3\sqrt 2}{\sqrt 3 \cdot \sqrt 6} = - 1[/imath]
[imath]\implies (\overrightarrow{a} , \overrightarrow{b}) = 180^\circ[/imath]

4.22. Tìm điều kiện của [imath]\overrightarrow{u}, \overrightarrow{v}[/imath] để:
a. [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath]
b. [imath]\overrightarrow{u} \cdot \overrightarrow{v} = - |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath]

Lời giải:
a.
Ta có: [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v} \cdot \cos(\overrightarrow{u}, \overrightarrow{u})[/imath]
Để [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath] thì [imath]\cos(\overrightarrow{u}, \overrightarrow{u}) = 1 \iff (\overrightarrow{u}, \overrightarrow{u}) = 0^\circ[/imath]
[imath]\implies \overrightarrow{u}, \overrightarrow{v}[/imath] cùng hướng
b.
Ta có: [imath]\overrightarrow{u} \cdot \overrightarrow{v} = |\overrightarrow{u}| \cdot |\overrightarrow{v} \cdot \cos(\overrightarrow{u}, \overrightarrow{u})[/imath]
Để [imath]\overrightarrow{u} \cdot \overrightarrow{v} = - |\overrightarrow{u}| \cdot |\overrightarrow{v}|[/imath] thì [imath]\cos(\overrightarrow{u}, \overrightarrow{u}) =- 1 \iff (\overrightarrow{u}, \overrightarrow{u}) = 180^\circ[/imath]
[imath]\implies \overrightarrow{u}, \overrightarrow{v}[/imath] ngược hướng

4.23. Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho hai điểm [imath]A(1;2), B(-4;3)[/imath]. Gọi [imath]M(t;0)[/imath] là một điểm thuộc trục hoành.
a. Tính [imath]\overrightarrow{AM} \cdot \overrightarrow{BM}[/imath] theo [imath]t[/imath]
b. Tính [imath]t[/imath] để [imath]\widehat{AMB} = 90^\circ[/imath]

Lời giải:
a. Ta có [imath]\overrightarrow{AM} = (t-1; -2), \overrightarrow{BM} = (t + 4; -3)[/imath]
[imath]\implies \overrightarrow{AM} \cdot \overrightarrow{BM} = (t - 1)(t+4) + (-2) (-3) = t^2 + 3t + 2[/imath]
b. Để [imath]\widehat{AMB} = 90^\circ[/imath] thì [imath]\overrightarrow{AM} \cdot \overrightarrow{AM} = 0[/imath]
[imath]\iff t^2 + 3t + 2 = 0 \iff \left[\begin{array}{l} t = -1 \\ t = -2 \end{array} \right.[/imath]

Timeless time4.24. Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho ba điểm không thẳng hàng [imath]A(-4;1), B(2;4), C(2;-2)[/imath].
a) Giải tam giác [imath]ABC[/imath].
b) Tìm tọa độ trực tâm [imath]H[/imath] của tam giác [imath]ABC[/imath].

GIải:
a. Ta có:
[imath]\overrightarrow{AB} = (6;3) \implies AB = 3\sqrt 5[/imath]
[imath]\overrightarrow{AC} = (6;-3) \implies AC = 3\sqrt 5[/imath]
[imath]\overrightarrow{BC} = (0;-6) \implies BC = 6[/imath]
Áp dụng định lý cosin ta có: [imath]\cos A = \dfrac{AC^2 + AB^2 - BC^2}{2 \cdot AB \cdot AC} = \dfrac{3}5[/imath]
[imath]\implies \hat A \approx 53,15^\circ[/imath]
Vì tam giác [imath]ABC[/imath] có [imath]AB = AC[/imath] nên suy ra [imath]\hat B = \hat C \approx 63,44^ \circ[/imath]
Vậy tam giác [imath]ABC[/imath] có : [imath]AB = AC = 3\sqrt 5, BC = 6 , \hat A \approx 53,15^\circ, \hat B = \hat C \approx 63,44^\circ[/imath]
b.
Gọi [imath]H (x;y)[/imath] là toạ độ trực tâm tam giác [imath]ABC[/imath].
Ta có: $
[imath]\overrightarrow{AH} (x+4; y - 1) , \overrightarrow{BC}(0;-6), \overrightarrow{BH} (x-2 ; y - 4), \overrightarrow{AC} (6;-3)[/imath]
Vì [imath]AH \perp BC \implies \overrightarrow{AH} \cdot \overrightarrow{BC} = 0 \iff (x + 4).0 + (y – 1).(–6) = 0 \iff y = 1[/imath]
Vì [imath]BH \perp AC \implies \overrightarrow{BH} \cdot \overrightarrow{AC} = 0 \iff (x – 2).6 + (y – 4).(–3) = 0 \iff x = \dfrac{1}2[/imath]
Vậy [imath]H \left (\dfrac{1}2;1 \right)[/imath]

4.25. Chứng minh rằng với mọi tam giác [imath]ABC[/imath], ta có: [imath]S_{ABC} = \dfrac{1}2 \sqrt{\overrightarrow{AB}^2 \cdot \overrightarrow{AC}^2 - (\overrightarrow{AB} \cdot \overrightarrow{AC})^2}[/imath].

Giải:
Ta có: [imath]S_{ABC} = \dfrac{1}2 AB \cdot AC \cdot \sin (\overrightarrow{AB} ; \overrightarrow{AC})[/imath]
[imath]= \dfrac{1}2 AB \cdot AC \sqrt{1 - \cos^2(\overrightarrow{AB} ; \overrightarrow{AC})}[/imath]
[imath]= \dfrac{1}2 AB \cdot AC \sqrt{1 - \cos \dfrac{(\overrightarrow{AB} \cdot \overrightarrow{AC})^2}{\overrightarrow{AB}^2 \cdot \overrightarrow{AC}^2 }}[/imath]
[imath]= \dfrac{1}2 AB \cdot AC \cdot \sqrt{\dfrac{\overrightarrow{AB}^2 \cdot \overrightarrow{AC}^2 - (\overrightarrow{AB} \cdot \overrightarrow{AC})^2}{\overrightarrow{AB}^2 \cdot \overrightarrow{AC}^2}}[/imath]
[imath]= \dfrac{1}2 \sqrt{\overrightarrow{AB}^2 \cdot \overrightarrow{AC}^2 - (\overrightarrow{AB} \cdot \overrightarrow{AC})^2}[/imath]

4.26. Cho tam giác [imath]ABC[/imath] có trọng tâm [imath]G[/imath]. Chứng minh rằng với mọi điểm [imath]M[/imath], ta có: [imath]MA^2 + MB^2 + MC^2 = 3MG^2 + GA^2 + GB^2 + GC^2[/imath].

Giải:
Ta có: [imath]MA^2 + MB^2 + MC^2 = \overrightarrow{MA}^2 + \overrightarrow{MB}^2 + \overrightarrow{MC}^2[/imath]
[imath]= (\overrightarrow{MG} + \overrightarrow{GA})^2 + (\overrightarrow{MG} + \overrightarrow{GB})^2 + (\overrightarrow{MG} + \overrightarrow{GC})^2[/imath]
[imath]= 3\overrightarrow{MG}^2 + 2\overrightarrow{MG} \cdot (\overrightarrow{GA} + \overrightarrow{GB} + \overrightarrow{GC}) + \overrightarrow{GA}^2 + \overrightarrow{GB}^2 + \overrightarrow{GC}^2[/imath]
Có: [imath]\overrightarrow{GA} + \overrightarrow{GB} + \overrightarrow{GC} = \overrightarrow{0}[/imath]
[imath]\implies \overrightarrow{MG} \cdot (\overrightarrow{GA} + \overrightarrow{GB} + \overrightarrow{GC}) = \overrightarrow{MG} \cdot \overrightarrow{0} = 0[/imath]
[imath]\implies MA^2 + MB^2 + MC^2 = 3\overrightarrow{MG}^2 + \overrightarrow{GA}^2 + \overrightarrow{GB}^2 + \overrightarrow{GC}^2[/imath]

 

[Timeless time]

MỘT SỐ DẠNG BÀI TẬP VỀ TÍCH VÔ HƯỚNG CỦA HAI VECTOR
​

Dạng 1: Chứng minh hai vector vuông góc
Dạng 2: Tìm [imath]m[/imath] để góc giữa hai vector bằng một số cho trước
Dạng 3: Tính độ dài vector, khoảng cách giữa hai điểm trong hệ tọa độ
Dạng: ......

DẠNG 1: CHỨNG MINH HAI VECTOR VUÔNG GÓC​

I. Phương pháp giải:
+ Phương pháp 1: Sử dụng định nghĩa
Nếu [imath](\overrightarrow{a}, \overrightarrow{b}) = 90^\circ[/imath] thì hai vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] vuông góc với nhau
+ Phương pháp 2: Sử dụng tính chất của tích vô hướng và áp dụng trong hệ tọa độ
Cho [imath]\overrightarrow{a}(x;y); \overrightarrow{b}(x';y')[/imath]
Khi đó: [imath]\overrightarrow{a} \perp \overrightarrow{b} \iff \overrightarrow{a} \cdot \overrightarrow{b} = 0\iff xx'+ yy' = 0[/imath]

II. Bài tập vận dụng

VD 1: Cho hai vector $\overrightarrow{a}$ và $\overrightarrow{b}$ vuông góc với nhau và $|\overrightarrow{a}| = 1$ và $|\overrightarrow{b}| = \sqrt 2$ . Chứng minh hai vector $2\overrightarrow{a} - \overrightarrow{b}$ và $\overrightarrow{a} + \overrightarrow{b}$ vuông góc với nhau.

Giải:
Do vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] vuông góc với nhau [imath]\implies \overrightarrow{a} \cdot \overrightarrow{b} =0[/imath]
Ta có: [imath]2\overrightarrow{a} - \overrightarrow{b} \cdot \overrightarrow{a} + \overrightarrow{b}= 2 \overrightarrow{a}^2 + 2\overrightarrow{a}\cdot \overrightarrow{b}- \overrightarrow{a}\cdot \overrightarrow{b} - \overrightarrow{b}^2[/imath]
[imath]= 2\overrightarrow{a}^2 + \overrightarrow{a} \cdot \overrightarrow{b} - \overrightarrow{b}^2[/imath]
[imath]= 2|\overrightarrow{a}|^2 + \overrightarrow{a} \cdot \overrightarrow{b} - |\overrightarrow{b}|^2[/imath]
[imath]= 2\cdot 1^2 + 0 - (\sqrt 2)^2[/imath]
[imath]=0[/imath]

VD 2: Cho tứ giác $ABCD$ có $ AB^2 + CD^2 = BC^2 + AD^2$. Chứng minh hai vector $\overrightarrow{DB}$ và $\overrightarrow{AC}$ vuông góc.

Giải:
[imath]A B^{2}+C D^{2}=B C^{2}+A D^{2}[/imath]
[imath]\Leftrightarrow \overrightarrow{A B}^{2}+\overrightarrow{C D}^{2}=\overrightarrow{B C}^{2}+\overrightarrow{A D}^{2}[/imath] (bình phương vô hướng bằng bình phương độ dài)
[imath]\Leftrightarrow \overrightarrow{A B}^{2}-\overrightarrow{A D}^{2}+\overrightarrow{C D}^{2}-\overrightarrow{B C}^{2}=0[/imath]
[imath]\Leftrightarrow(\overrightarrow{A B}-\overrightarrow{A D})(\overrightarrow{A B}+\overrightarrow{A D})+(\overrightarrow{C D}-\overrightarrow{B C})(\overrightarrow{C D}+\overrightarrow{B C})=\overrightarrow{0}[/imath]
[imath]\Leftrightarrow \overrightarrow{D B}(\overrightarrow{A B}+\overrightarrow{A D})+(\overrightarrow{C D}-\overrightarrow{B C}) \overrightarrow{B D}=\overrightarrow{0}[/imath] (quy tắc ba điểm)
[imath]\Leftrightarrow \overrightarrow{D B}(\overrightarrow{A B}+\overrightarrow{A D})-\overrightarrow{D B}(\overrightarrow{C D}-\overrightarrow{B C})=\overrightarrow{0}[/imath] (vectơ đối)
[imath]\Leftrightarrow \overrightarrow{D B}(\overrightarrow{A B}+\overrightarrow{A D}-\overrightarrow{C D}+\overrightarrow{B C})=\overrightarrow{0}[/imath]
[imath]\Leftrightarrow \overrightarrow{D B}(\overrightarrow{A B}+\overrightarrow{B C}+\overrightarrow{A D}+\overrightarrow{D C}[/imath] ) [imath]=\overrightarrow{0}[/imath] (quy tắc ba điểm)
[imath]\Leftrightarrow \overrightarrow{D B}(\overrightarrow{A C}+\overrightarrow{A C})=\overrightarrow{0}[/imath]
[imath]\Leftrightarrow \overrightarrow{D B} \cdot 2 \overrightarrow{A C}=0[/imath]
[imath]\Leftrightarrow \overrightarrow{D B} \cdot \overrightarrow{A C}=0[/imath]
Vậy [imath]\overrightarrow{D B} \perp \overrightarrow{A C}[/imath].

III. Bài tập tự luyện
Bài 1: Cho tam giác [imath]ABC[/imath] vuông tại [imath]A[/imath] có [imath]AB = a, AC = 2a[/imath]. Gọi M là trung điểm của [imath]BC[/imath] và điểm [imath]D[/imath] bất kỳ thuộc cạnh [imath]AC[/imath]. Tính [imath]AD[/imath] theo [imath]a[/imath] để [imath]BD \perp AM[/imath].
Bài 2: Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho vector [imath]\overrightarrow{a}=(9;3)[/imath]. Vectơ nào sau đây không vuông góc với vector [imath]\overrightarrow{a}[/imath].
A. [imath]\overrightarrow{b}= (1;-3)[/imath]
B. [imath]\overrightarrow{b}= (2;-6)[/imath]
C. [imath]\overrightarrow{b}=(1;3)[/imath]
D. [imath]\overrightarrow{b}=(-1;3)[/imath]
Bài 3: Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho hai vector [imath]\overrightarrow{u} = \dfrac{1}2 \overrightarrow{i} - 5\overrightarrow{j}[/imath] và [imath]\overrightarrow{v} = k\overrightarrow{i} - 4\overrightarrow{b}[/imath] . Tìm [imath]k[/imath] để hai vector [imath]\overrightarrow{u}[/imath] và [imath]\overrightarrow{v}[/imath] vuông góc với nhau.
A. [imath]k = 20[/imath]
B. [imath]k = 40[/imath]
C. [imath]k = -20[/imath]
D. [imath]k = -40[/imath]

 

[Timeless time]

DẠNG 2: TÌM M ĐỂ GÓC GIỮA HAI VECTOR BẰNG MỘT SỐ CHO TRƯỚC​

I. Phương pháp giải:
Các bước làm bài:
Bước 1: Xác định vector (nếu chưa có) theo tham số [imath]m[/imath]
Bước 2: Tính độ dài các vector theo tham số [imath]m[/imath]
Bước 3: Áp dụng công thức tính [imath]\cos[/imath] góc giữa hai vector
Trong hệ toạ độ cho hai vector [imath]\overrightarrow{a} = (a_1;a_2)[/imath] và [imath]\overrightarrow{b} = (b_1;b_2)[/imath]
[imath]\cos (\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|} =\dfrac{a_1b_1 + a_2b_2}{\sqrt{a_1^2 + a_2^2} + \sqrt{b_1^2 + b_2^2}}[/imath]
Bước 4: Đưa ra phương trình chứa ẩn [imath]m[/imath]
Góc giữa hai vector bằng [imath]\alpha \iff \cos (\overrightarrow{a}, \overrightarrow{b}) = \cos \alpha[/imath]
Bước 5: Giải phương trình đưa ra giá trị của [imath]m[/imath]

II. Bài tập vận dụng
Bài 1: Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho hai vector [imath]\overrightarrow{a}= (3;m)[/imath] và [imath]\overrightarrow{b}= (1;7)[/imath]. Xác định [imath]m[/imath] để góc giữa hai vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] là [imath]45^\circ[/imath]
Giải:
Ta có: [imath]\cos(\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|} = \dfrac{3 \cdot 1 + 7 \cdot m}{\sqrt{3^2 + m^2} + \sqrt{1^2 + 7^2}} = \dfrac{3 + 7m}{ \sqrt{m^2 + 9}\cdot \sqrt{50}}[/imath]
Có góc giữa hai vector [imath]\overrightarrow{a}, \overrightarrow{b} = 45^\circ \implies \cos (\overrightarrow{a}, \overrightarrow{b}) = \cos 45^\circ[/imath]
[imath]\iff \dfrac{3 + 7m}{m^2 + 9 \sqrt{50}} = \dfrac{\sqrt 2}2[/imath]
[imath]\iff 2(3+ 7m) = \sqrt 2 \cdot \sqrt{m^2 + 9} \cdot \sqrt{50}[/imath]
[imath]\iff 5\sqrt{m^2 + 9} = 7m + 3[/imath]
[imath]\iff \begin{cases} 7m + 3 \ge 0 \\ ( 5\sqrt{m^2 + 9})^2 = (7m +3)^2 \end{cases}[/imath]
[imath]\iff \begin{cases} m \ge - \dfrac{3}7 \\ 25(m^2 + 9) = 49^2 + 42m + 9 \end{cases}[/imath]
[imath]\iff \dots[/imath]
[imath]\implies m = \dfrac{9}4[/imath]

Bài 2: Trong mặt phẳng tọa độ Oxy cho hai vector [imath]\overrightarrow{u} = (4;1)[/imath] và vector [imath]\overrightarrow{v} = (1;4)[/imath]. Tìm [imath]m[/imath] để vector [imath]\overrightarrow{a}=m.\overrightarrow{u} + \overrightarrow{v}[/imath] tạo với vector [imath]\overrightarrow{b} =\overrightarrow{i}+\overrightarrow{j}[/imath] một góc [imath]45^\circ[/imath].
Giải:
Ta có:
[imath]\overrightarrow{a} = m \cdot \overrightarrow{u} + \overrightarrow{v} = (4m +1; m+ 4)[/imath]
[imath]\overrightarrow{b} = \overrightarrow{i} + \overrightarrow{j} = (1;1)[/imath]
Có: [imath]\cos(\overrightarrow{a}, \overrightarrow{b}) = \dfrac{\overrightarrow{a} \cdot \overrightarrow{b}}{|\overrightarrow{a}| \cdot |\overrightarrow{b}|}[/imath]
[imath]= \dfrac{(4m+1)\cdot 1 + (m+ 4)\cdot 1}{\sqrt{(4m+ 1)^2 + (m + 4)^2}\cdot \sqrt{1^2 +1^2}}[/imath]
[imath]= \dfrac{5m + 5}{\sqrt{17m^2 + 16m + 17}\cdot \sqrt 2}[/imath]
Có góc giữa hai vector [imath]\overrightarrow{a}, \overrightarrow{b} = 45^\circ \implies \cos (\overrightarrow{a}, \overrightarrow{b}) = \cos 45^\circ[/imath]
[imath]\iff \dfrac{5m + 5}{\sqrt{17m^2 + 16m + 17}\cdot \sqrt 2} = \dfrac{\sqrt 2}2[/imath]
[imath]\iff 2(5m+5) = \sqrt 2 \cdot \sqrt{17m^2 + 16m + 17}\cdot \sqrt 2[/imath]
[imath]\iff \begin{cases} 2(5m +5) \ge 0 \\ (2\cdot (5m +5))^2 = (\sqrt 2 \cdot \sqrt{17m^2 + 16m + 17}\cdot \sqrt 2)^2 \end{cases}[/imath]
[imath]\iff \dots[/imath]
[imath]\iff \begin{cases} m \ge 1 \\ 8m^2 + 34m + 8 = 0 \end{cases}[/imath]
[imath]\implies m = -\dfrac{1}4[/imath]

III. Bài tập tự luyện
Bài 1: Tìm m để hai vector [imath]\overrightarrow{a} =(1;-3);\overrightarrow{b} =(m^2;4)[/imath] tạo với nhau một góc bằng [imath]90^\circ[/imath]
A. [imath]m = 12[/imath]
B. [imath]m = 2\sqrt 3[/imath]
C. [imath]m = -2\sqrt 3[/imath]
D. [imath]m = \pm 2\sqrt 3[/imath]
Bài 2: Cho hai vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] thỏa mãn các điều kiện: [imath]|\overrightarrow{a}| = \dfrac{1}2 |\overrightarrow{b}| = 1; |\overrightarrow{a} - 2\overrightarrow{b}| = \sqrt{15}[/imath]
Đặt [imath]\overrightarrow{u} = \overrightarrow{a} + \overrightarrow{b}[/imath] và [imath]\overrightarrow{v}= 2k \overrightarrow{a} - \overrightarrow{b} \ \ k \in \mathbb R[/imath]. Tìm tất cả giá trị của k sao cho [imath](\overrightarrow{u};\overrightarrow{v}) = 60^\circ[/imath]
A. [imath]4 + \dfrac{3\sqrt 5}{2}[/imath]
B. [imath]4\pm \dfrac{3\sqrt 5}{2}[/imath]
C. [imath]5 + \dfrac{\sqrt 17}2[/imath]
D. [imath]5 \pm \dfrac{\sqrt 17}2[/imath]
Bài 3: Trong mặt phẳng tọa độ [imath]Oxy[/imath], cho hai vector [imath]\overrightarrow{a} = (3;m)[/imath] và [imath]\overrightarrow{b} = (1;7)[/imath]. Xác định [imath]m[/imath] để góc giữa hai vector [imath]\overrightarrow{a}[/imath] và [imath]\overrightarrow{b}[/imath] là [imath]45^\circ[/imath].