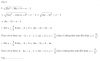Nhờ mọi người giúp mình. Cảm ơn ạ!
Câu 2:
a) ĐK: $x\ge -3$
pt $\Leftrightarrow 2\sqrt{x+3}-3\sqrt{x+3}-2=\sqrt{x+3}$
$\Leftrightarrow 2\sqrt{x+3}=-2$
$\Leftrightarrow \sqrt{x+3}=-1$ (vô lí)
Vậy pt vô nghiệm
Câu 4:
a) $P=(\dfrac{2\sqrt x}{\sqrt x+3}+\dfrac{\sqrt x}{\sqrt x-3}+\dfrac{3x+3}{9-x})(\dfrac{\sqrt x-7}{\sqrt x+1}+1)$
$=\dfrac{2\sqrt x(\sqrt x-3)+\sqrt x(\sqrt x+3)-(3x+3)}{(\sqrt x-3)(\sqrt x+3)}.\dfrac{\sqrt x-7+\sqrt x+1}{\sqrt x+1}$
$=\dfrac{2x-6\sqrt x+x+3\sqrt x-3x-3}{(\sqrt x-3)(\sqrt x+3)}.\dfrac{2\sqrt x-6}{\sqrt x+1}$
$=\dfrac{-3(\sqrt x+1)}{(\sqrt x-3)(\sqrt x+3)}.\dfrac{2(\sqrt x-3)}{\sqrt x+1}$
$=\dfrac{-6}{\sqrt x+3}$
b) $P\ge \dfrac{-1}2\Leftrightarrow \dfrac{-6}{\sqrt x+3}+\dfrac{1}2\ge 0\Leftrightarrow \dfrac{\sqrt x-9}{2(\sqrt x+3)}\ge 0\Leftrightarrow \sqrt x-9\ge 0\Leftrightarrow x\ge 81$ (TM)
Vậy với $\ge 81$ thì $P\ge \dfrac{-1}2$
c) Ta có: $\sqrt x+3\ge 3\Rightarrow \dfrac{-6}{\sqrt x+3}\ge \dfrac{-6}3=-2$
Dấu '=' xảy ra khi $x=0$ (TM)
Vậy $P_{min}=-2$ khi $x=0$
d) Với $x=-7\sqrt[3]{49(5+4\sqrt 2)(3+2\sqrt{1+\sqrt 2})(3-2\sqrt{1+\sqrt 2})}$
$=-7\sqrt[3]{49(5+4\sqrt 2)[9-4(1+\sqrt 2)]}$
$=-7\sqrt[3]{49(5+4\sqrt 2)(5-4\sqrt 2)}$
$=-7\sqrt[3]{49(25-32)}$
$=-7\sqrt[3]{-343}=49$ (TM)
Thì giá trị của biểu thức $P$ là: $P=\dfrac{-6}{\sqrt{49}+3}=\dfrac{-6}{10}=\dfrac{-3}5$
 [TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
 Chắc suất Đại học top - Giữ chỗ ngay!!
ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.
Chắc suất Đại học top - Giữ chỗ ngay!!
ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.