[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
 Chắc suất Đại học top - Giữ chỗ ngay!!
ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.
Chắc suất Đại học top - Giữ chỗ ngay!!
ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.
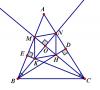
cho tam giác nhọn ABC , đường cao BD , CE , tia phân giác của các góc ABD và ACE cắt nhau tại O , cắt AC và AB lần lượt tại N và M . tia BN cắt CE tại K , tia CM cắt BD tại H . chứng minh :
a , BN vuông góc CM
b, MNHK là hình thoi
a , BN vuông góc CM
b, MNHK là hình thoi