View attachment 160775
mọi người vẽ véc tơ lực chiếu hình và làm cụ thể mình với
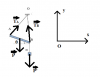
Chọn hệ trục Oxy như hình vẽ. Để hệ cân bằng thì:
[tex]\vec{T_A}+\vec{T_B}+\vec{P}+\vec{P'}=\vec{0}[/tex]
Chiếu lên phương Ox:
[tex]T_A.cos(60^0-\theta)-T_{B}.sin(30^0-\theta)=0[/tex]
[tex]\Rightarrow T_A.cos(60^0-\theta).cos(30^0-\theta)-T_{B}.sin(30^0-\theta)cos(30^0-\theta)=0[/tex] (1)
Chiếu lên phương Oy:
[tex]T_A.sin(60^0-\theta)+T_B.cos(30^0-\theta)-2mg=0[/tex]
[tex]\Rightarrow T_A.sin(60^0-\theta).sin(30^0-\theta)+T_B.cos(30^0-\theta).sin(30^0-\theta)-2mg.sin(30^0-\theta)=0[/tex] (2)
Chọn trục quay tại B, hệ đứng yên nên:
[tex]\frac{1}{2}mgL.cos\theta-T_AL.sin60^0=0\Leftrightarrow \frac{1}{2}mgL.cos\theta=T_AL.sin60^0[/tex][tex]\Rightarrow \frac{1}{2}mg.cos\theta=T_A.sin60^0[/tex] (3)
Lấy (1) cộng với (2) suy ra: [tex]T_A.cos30^0=2mg.sin(30^0-\theta)[/tex] (4)
Lấy (4) chia (3) ta được: [tex]1=4.\frac{sin(30^0-\theta)}{cos\theta}\Rightarrow \theta\approx 16^06'[/tex]
Có [tex]\theta[/tex] rồi thì thay vào (4) và (1) là ra [tex]T_A[/tex] và [tex]T_B[/tex] thôi
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
