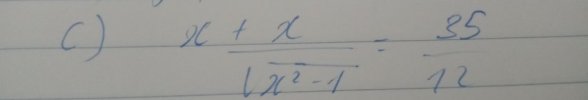[imath]x+\dfrac{x}{\sqrt{x^2-1}}=\dfrac{35}{12}[/imath]
ĐKXĐ [imath]\left[\begin{matrix}x>1\\x<-1\end{matrix}\right.[/imath]
Nếu [imath]x<0[/imath] thì VT<0<VP (loại)
Xét [imath]x>1[/imath] ta có
Đặt [imath]x=\dfrac{1}{\sin t} \quad t\in\left(0, \dfrac{\pi}2\right)[/imath]
[imath]\Rightarrow \sqrt{x^2-1}=\sqrt{\dfrac{1}{\sin^2t}-1}=\sqrt{\dfrac{1-\sin^2t}{\sin^2t}}=\sqrt{\dfrac{\cos ^2t}{\sin^2t}}=\dfrac{\cos t}{\sin t}[/imath]
PT tt: [imath]\dfrac{1}{\sin t}+\dfrac{1}{\sin t}.\dfrac{\sin t}{\cos t}=\dfrac{35}{12}[/imath]
[imath]\Rightarrow \dfrac{1}{\sin t}+\dfrac{1}{\cos t}=\dfrac{35}{12}\Rightarrow \sin t+\cos t=\dfrac{35}{12}\sin t\cos t[/imath] (*)
Đặt [imath]a=\sin t+\cos t\Rightarrow a^2=1+2\cos t\sin t\Rightarrow \cos t\sin t=\dfrac{a^2-1}2[/imath]
Em thay vào pt (*) để giải tiếp nha
Có gì khúc mắc em hỏi lại nha
Ngoài ra, em xem thêm tại
[Lý thuyết] Chuyên đề HSG: Phương trình vô tỷ
[Bài tập] Chuyên đề HSG: Phương trình vô tỷ
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
