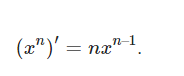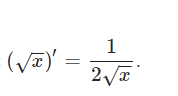Toán 11 Chứng minh công thức đạo hàm

[Andy3012]

[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
Chắc suất Đại học top - Giữ chỗ ngay!!

ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.

Chứng minh các công thức sau
[imath](x^n)'=nx^{n-1}[/imath]
[imath](\sqrt{x})'=\dfrac{1}{2\sqrt{x}}[/imath]
[imath](\dfrac{1}{x})'=\dfrac{-1}{x^2}[/imath]
[imath](\cos x)'=-\sin x[/imath]
Giúp em với ạ
Em cảm ơn mn nhiều

 

[Alice_www]

Chứng minh các công thức sau
[imath](x^n)'=nx^{n-1}[/imath]
[imath](\sqrt{x})'=\dfrac{1}{2\sqrt{x}}[/imath]
[imath](\dfrac{1}{x})'=\dfrac{-1}{x^2}[/imath]
[imath](\cos x)'=-\sin x[/imath]
Giúp em với ạ
Em cảm ơn mn nhiều

Andy3012
a) [imath]f(x)=x^n[/imath]
[imath]f'(x)=\lim \limits_{t\to 0} \dfrac{f(x+t)-f(x)}{t}=\lim \limits_{t\to 0} \dfrac{(x+t)^n-x^n}{t}[/imath]
[imath]=\lim \limits_{t\to 0} \dfrac{(x+t-x)[(x+t)^{n-1}+(x+t)^{n-2}x+...+x^{n-1}]}{t}=nx^{n-1}[/imath]
b) [imath]f(x)=\sqrt{x}[/imath]
[imath]f'(x)= \lim \limits_{t\to 0} \dfrac{f(x+t)-f(x)}{t}=\lim \limits_{t\to 0} \dfrac{\sqrt{x+t}-\sqrt{x}}{t}[/imath]
[imath]=\lim \limits_{t\to 0} \dfrac{x+t-x}{(\sqrt{x+t}+\sqrt{x})t}=\lim \limits_{t\to 0} \dfrac{1}{\sqrt{x+t}+\sqrt{t}}=\dfrac{1}{2\sqrt{x}}[/imath]
Hai câu còn lại em thử làm tương tự nhé, nếu không được em đăng thành chủ đề mới để được hỗ trợ nha
Có gì khúc mắc em hỏi lại nhé
Ngoài ra em tham khảo thêm tại Tổng hợp kiến thức toán 11