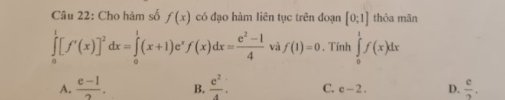[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
 Chắc suất Đại học top - Giữ chỗ ngay!!
ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.
Chắc suất Đại học top - Giữ chỗ ngay!!
ĐĂNG BÀI NGAY để cùng trao đổi với các thành viên siêu nhiệt tình & dễ thương trên diễn đàn.
Câu 22: Cho hàm số f(x) có đạo hàm liên tục trên đoạn [0;1] thỏa mãn
[imath]\displaystyle \int_{0}^{1}\left[f^{\prime}(x)\right]^{2} \mathrm{~d} x=\displaystyle \int_{0}^{1}(x+1) \mathrm{e}^{x} f(x) \mathrm{d} x=\dfrac{\mathrm{e}^{2}-1}{4}[/imath] và [imath]f(1)=0[/imath]. Tính [imath] \displaystyle \int_{0}^{1} f(x) \mathrm{d} x[/imath]
[imath]\displaystyle \int_{0}^{1}\left[f^{\prime}(x)\right]^{2} \mathrm{~d} x=\displaystyle \int_{0}^{1}(x+1) \mathrm{e}^{x} f(x) \mathrm{d} x=\dfrac{\mathrm{e}^{2}-1}{4}[/imath] và [imath]f(1)=0[/imath]. Tính [imath] \displaystyle \int_{0}^{1} f(x) \mathrm{d} x[/imath]
Attachments
Last edited by a moderator: