Cho hình chóp S ABC . có đáy ABC là tam giác vuông tại B, AB =1 và AC = 2. Cạnh bên SA vuông góc với mặt phẳng đáy. Góc giữa hai mặt phẳng (SAC)và (SBC)là 60 .Thể tích khối chóp S ABC bằng?
Nhờ anh chị giải giúp em bài này với ạ. em cảm ơn anh chị
hihihahahoneyxx.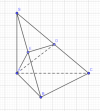
Kẻ [imath]AE\bot SB, AD\bot SC[/imath]
Ta có: [imath]CB\bot AB, CB\bot SA\Rightarrow CB\bot (SAB)\Rightarrow CB\bot AE[/imath]
Suy ra [imath]AE\bot (SBC)[/imath]
[imath]\Rightarrow AE\bot SC[/imath]
Mà [imath]SC\bot AD[/imath]
Suy ra [imath]SC\bot (AED)[/imath]
[imath]\Rightarrow ((SAC),(SBC))=\widehat{ADE}[/imath]
[imath]AE\bot (SBC)\Rightarrow AE\bot ED[/imath]
[imath]\Rightarrow \sin \widehat{ADE}=\dfrac{AE}{AD}=\dfrac{\sqrt3}2[/imath]
Đặt [imath]SA=a[/imath]
Ta có: [imath]\dfrac{1}{AD^2}=\dfrac{1}{SA^2}+\dfrac1{AC^2}=\dfrac{1}{a^2}+\dfrac{1}4\Rightarrow AD=\dfrac{2a}{\sqrt{4+a^2}}[/imath]
[imath]\dfrac{1}{AE^2}=\dfrac{1}{SA^2}+\dfrac{1}{AB^2}=\dfrac{1}{a^2}+1\Rightarrow AE=\dfrac{a}{\sqrt{a^2+1}}[/imath]
Suy ra [imath]\dfrac{\sqrt{4+a^2}}{2\sqrt{a^2+1}}=\dfrac{\sqrt3}2\Rightarrow a=\dfrac{\sqrt2}2[/imath]
[imath]S_{S.ABC}=\dfrac{1}3SA.\dfrac{1}2AB.BC=\dfrac{\sqrt6}{12}[/imath]
Có gì khúc mắc em hỏi lại nhé
Ngoài ra em xem thêm tại
Đường thẳng và mặt phẳng trong không gian. Quan hệ song song
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
