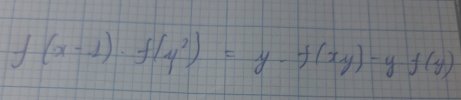Ký hiệu [imath]P(x,y)[/imath] là phép thế cặp [imath](x,y)[/imath] vào đề bài.
[imath]P(1,1) \Rightarrow f(0)f(1)=0[/imath]
[imath]\Rightarrow \left[\begin{array}{l} f(0)=0 \\ f(1)=0 \end{array}\right.[/imath]
Nếu [imath]f(1)=0[/imath] thì [imath]P(x,1) \Rightarrow f(x)=0 \forall x \in \mathbb{Z}[/imath]
Nếu [imath]f(0)=0[/imath].
[imath]P(0,1) \Rightarrow f(-1)f(1)=-f(1)[/imath]
[imath]\Rightarrow \left[\begin{array}{l} f(-1)=-1 \\ f(1)=0 \end{array}\right.[/imath]
Với [imath]f(1)=0[/imath] thì như trên ta có [imath]f(x)=0 \forall x \in \mathbb{Z}[/imath]
Với [imath]f(-1)=-1[/imath] thì [imath]P(0,x) \Rightarrow -f(x^2)=-xf(x) \forall x \in \mathbb{Z}[/imath]
[imath]\Rightarrow f(x^2)=xf(x) \forall x \in \mathbb{Z}[/imath]
Thay [imath]x[/imath] bởi [imath]-x[/imath] vào đẳng thức trên ta có [imath]f(x^2)=-xf(-x)[/imath]
[imath]\Rightarrow xf(x)=-xf(-x) \forall x \in \mathbb{Z} \Rightarrow f(-x)=-f(x) \forall x \in \mathbb{Z}, x \neq 0[/imath]
Mà [imath]f(0)=0[/imath] nên ta có [imath]f[/imath] là hàm lẻ.
[imath]\Rightarrow f(1)=-f(-1)=1[/imath]
[imath]P(x,1) \Rightarrow f(x-1)=f(x)-1[/imath]
Từ đó bằng quy nạp ta thấy [imath]f(x)=x[/imath]. Thử lại ta thấy thỏa mãn.
Vậy có [imath]2[/imath] hàm thỏa mãn đề bài là [imath]f(x)=x \forall x \in \mathbb{Z}[/imath] và [imath]f(x)=0 \forall x \in \mathbb{Z}[/imath]
Nếu còn thắc mắc chỗ nào bạn hãy trả lời dưới topic này để được hỗ trợ nhé ^^ Chúc bạn học tốt ^^
Ngoài ra, bạn tham khảo kiến thức tại đây nhé
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
