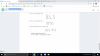
17. Giải hệ phương trình: $\left\{\begin{matrix} \dfrac1x+\dfrac1y=\dfrac{3}4\\\dfrac1{6x}+\dfrac1{5y}=\dfrac{2}{15} \end{matrix}\right.$
18. Giải hệ phương trình: $\left\{\begin{matrix} x^2+y^3=5\\2x^2-3y^3=5 \end{matrix}\right.$
17. ĐK: $x\ne 0;y\ne 0$
Đặt $a=\dfrac1x;b=\dfrac1y$
$hpt\Leftrightarrow \left\{\begin{matrix} a+b=\dfrac{3}4\\\dfrac16a+\dfrac15b=\dfrac{2}{15} \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a+b=\dfrac{3}4\\a+\dfrac{6}5b=\dfrac{4}{5} \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a+b=\dfrac{3}4\\\dfrac15b=\dfrac{1}{20} \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a+b=\dfrac{3}4\\b=\dfrac{1}{4} \end{matrix}\right.\\\Leftrightarrow \left\{\begin{matrix} a=\dfrac{1}2\\b=\dfrac{1}{4} \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} \dfrac1x=\dfrac12\\\dfrac1y=\dfrac{1}{4} \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x=2\\y=4 \end{matrix}\right.$
Vậy hệ phương trình có 1 cặp nghiệm $(x;y)=(2;4)$.
18.
Đặt $a=x^2;b=y^3$
$hpt\Leftrightarrow \left\{\begin{matrix} a+b=5\\2a-3b=5 \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} 3a+3b=15\\2a-3b=5 \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} 5a=20\\a+b=5 \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a=4\\a+b=5 \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} a=4\\b=1 \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x^2=4\\y^3=1 \end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x=\pm 2\\y=1 \end{matrix}\right.$
Vậy hệ phương trình có 2 cặp nghiệm $(x;y)=(-2;1)$ và $(x;y)=(2;1)$
Bài còn lại bạn đăng chủ đề mới để được hỗ trợ nhé. Hãy đăng bài theo
NỘI QUY BOX TOÁN để được hỗ trợ sớm nhất nhé.
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
