[imath]g'(x)=f'(f^2(x)-3f(x)-m)\cdot [2f(x)f'(x)-3f'(x)]=f'(f^2(x)-3f(x)-m)\cdot f'(x)[2f(x)-3][/imath]
Để [imath]g(x)[/imath] có ít nhất [imath]13[/imath] điểm cực trị thì [imath]g'(x)[/imath] có ít nhất [imath]13[/imath] nghiệm phân biệt.
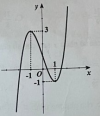
Ta thấy [imath]f(x)[/imath] có [imath]2[/imath] điểm cực trị là [imath](-1,3);(1,-1)[/imath] nên [imath]f'(x)=0[/imath] có [imath]2[/imath] nghiệm [imath]x=-1 \vee x=1[/imath]
[imath]2f(x)-3=0 \Leftrightarrow f(x)=\dfrac{3}{2}[/imath]. Phương trình [imath]f(x)=\dfrac{3}{2}[/imath] có [imath]3[/imath] nghiệm phân biệt.
Đặt [imath]h(x)=x^2-3x[/imath].
[imath]f'(h(f(x))-m)=0 \Leftrightarrow h(f(x))-m=-1 \vee h(f(x))-m=1[/imath]
[imath]\Leftrightarrow h(f(x))=m-1(1) \vee h(f(x))=m+1(2)[/imath]
Nhận thấy [imath](1),(2)[/imath] có tối đa [imath]6[/imath] nghiệm nên để [imath]g'(x)=0[/imath] có ít nhất [imath]13[/imath] nghiệm phân biệt thì [imath](1),(2)[/imath] đều phải có ít nhất [imath]2[/imath] nghiệm.
Vì [imath](1),(2)[/imath] đều có nghiệm nên ta xét [imath]m -1 \geq -\dfrac{9}{4} \Leftrightarrow m \geq \dfrac{-5}{4}[/imath]
Xét bảng biến thiên của [imath]h(f(x))[/imath]
[math]\begin{array}{c|ccccccccccccc} x & -\infty & & x_1 & & -1 & & x_2 & & 1 & & x_3 & & +\infty \\ \hline & & & & & 3 & & & & & & & & +\infty \\ & & & & \nearrow & & \searrow & & & & & & \nearrow & \\ & & & \dfrac{3}{2} & & & & \dfrac{3}{2} & & & & \dfrac{3}{2} & & \\ & & \nearrow & & & & & & \searrow & & \nearrow & & & \\ f(x) & -\infty & & & & & & & & -1 & & & & \\ \hline h(f(x)) & +\infty & & & & 0 & & & & 4 & & & & +\infty \\ & & \searrow & & \nearrow & & \searrow & & \nearrow & & \searrow & & \nearrow & \\ & & & -\dfrac{9}{4} & & & & -\dfrac{9}{4} & & & & -\dfrac{9}{4} & & \end{array}[/math]Xét các trường hợp:
+ [imath]m=-\dfrac{5}{4}[/imath]
Từ bảng biến thiên thì ta thấy [imath](1) \Leftrightarrow f(x)=\dfrac{3}{2}[/imath] nên ta không xét tiếp các nghiệm của (1).
[imath](2) \Leftrightarrow h(f(x))=-\dfrac{1}{4} \Leftrightarrow f(x)=\dfrac{-2\sqrt{2}+3}{2} \in (0,1) \vee f(x)=\dfrac{2\sqrt{2}+3}{2} \in (2,3)[/imath]
Với mỗi phương trình trên ta nhận được [imath]3[/imath] nghiệm [imath]x[/imath].
Từ đó số nghiệm của [imath]g'(x)=0[/imath] là [imath]2+3+3+3=11[/imath] (loại)
+ [imath]m \in (-\dfrac{5}{4},-1)[/imath]
Khi đó [imath](1),(2)[/imath] đều có [imath]6[/imath] nghiệm và khác với [imath]5[/imath] nghiệm trước đó của [imath]g'(x)=0[/imath] nên [imath]g'(x)=0[/imath] có [imath]2+3+6+6=17[/imath] nghiệm (thỏa mãn)
+ [imath]m=-1[/imath]
Khi đó [imath](1)[/imath] có [imath]6[/imath] nghiệm còn [imath](2) \Leftrightarrow \left[\begin{array}{l} x=-1 \\ x=t_1 \in (\infty,x_1) \\ x=t_2 \in (x_2,1) \\ x=t_3 \in (1,x_3) \\ x=t_4 \in (x_3,+\infty) \end{array}\right.[/imath]
Từ đó [imath]g'(x)=0[/imath] có [imath]2+3+6+4=15[/imath] nghiệm (thỏa mãn)
+ [imath]m \in (-1,1)[/imath]
Khi đó [imath](1)[/imath] có [imath]6[/imath] nghiệm, [imath](2)[/imath] có [imath]4[/imath] nghiệm nên thỏa mãn.
+ [imath]m =1[/imath]
Khi đó [imath](1) \Leftrightarrow \left[\begin{array}{l} x=-1 \\ x=t_1 \\ x=t_2 \\ x=t_3 \\ x=t_4 \end{array}\right.[/imath] và [imath](2)[/imath] có [imath]4[/imath] nghiệm nên [imath]g'(x)=0[/imath] có [imath]2+3+4+4=13[/imath] nghiệm (thỏa mãn)
+ [imath]m \in (1,3)[/imath]
Khi đó [imath](1),(2)[/imath] đều có [imath]4[/imath] nghiệm nên thỏa mãn.
+ [imath]m =3[/imath]
Khi đó [imath](1)[/imath] có [imath]4[/imath] nghiệm và [imath](2) \Leftrightarrow \left[\begin{array}{l} x=1 \\ x=t_5 \in (\infty,x_1) \\ x=t_6 \in (x_3,+\infty) \end{array}\right.[/imath] nên [imath]g'(x)=0[/imath] có [imath]2+3+4+2=11[/imath] nghiệm (không thỏa mãn)$
+ [imath]m >3[/imath]
Khi này thì [imath](1),(2)[/imath] sẽ có tổng số nghiệm không quá số nghiệm của [imath](1),(2)[/imath] tại [imath]m=3[/imath] nên không thỏa mãn.
Từ đó [imath]m \in (-\dfrac{5}{4},3)[/imath] nên có [imath]4[/imath] số nguyên [imath]m[/imath] thỏa mãn đề bài.
Nếu còn thắc mắc chỗ nào bạn hãy trả lời dưới topic này để được hỗ trợ nhé. Chúc bạn học tốt ^^
Ngoài ra, bạn tham khảo kiến thức tại topic này nha
Chinh phục kì thi THPTQG môn Toán 2022
[TẶNG BẠN] TRỌN BỘ Bí kíp học tốt 08 môn
